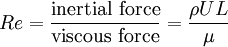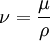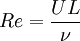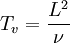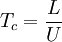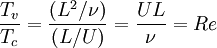Reynolds number
From CFD-Wiki
The Reynolds number characterises the relative importance of inertial and viscous forces in a flow. It is important in determining the state of the flow, whether it is laminar or turbulent. At high Reynolds numbers flows generally tend to be turbulent, which was first recognized by Osborne Reynolds in his famous pipe flow experiments. Consider the momentum equation which is given below
The terms on the right are the inertial forces and those on the left correspond to viscous forces. If 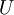 ,
, 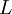 ,
, 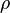 and
and 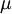 are the reference values for velocity, length, density and dynamic viscosity, then
are the reference values for velocity, length, density and dynamic viscosity, then
inertial force ~ 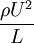
viscous force ~ 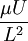
Their ratio is the Reynolds number, usually denoted as 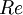
In terms of the kinematic viscosity
the Reynolds number is given by
Reynolds number as a ratio of time scales
Consider an impulsively started flat plate moving in its own plane with velocity 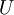 . Due to the no-slip condition on the plate a boundary layer gradually develops on the plate. At time
. Due to the no-slip condition on the plate a boundary layer gradually develops on the plate. At time 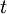 , the thickness of the boundary layer is of the order of
, the thickness of the boundary layer is of the order of 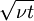 (see Batchelor(1967), section 4.3). Let
(see Batchelor(1967), section 4.3). Let 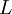 be the characteristic length scale. The time taken for viscous and convective effects to travel a distance
be the characteristic length scale. The time taken for viscous and convective effects to travel a distance 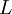 is
is
and
The ratio of viscous to convective time scales is
Thus the Reynolds number is a measure of the viscous and convective time scales. A large Reynolds number means that viscous effects propagate slowly into the fluid. This is the reason why boundary layers are thin in high Reynolds number flows because the fluid is being convected along the flow direction at a much faster rate than the spreading of the boundary layer, which is normal to the flow direction.
References
- Batchelor, G K (1967), An Introduction to Fluid Dynamics, Cambridge University Press.
- Rott, N (1990), Note on the history of the Reynolds number, Annual Review of Fluid Mechanics, Vol. 22, 1990, pp. 1–11.

![\frac{\partial}{\partial t}\left( \rho u_i \right) +
\frac{\partial}{\partial x_j}
\left[ \rho u_i u_j + p \delta_{ij} \right] = \frac{\partial}{\partial x_j} \tau_{ij}](/W/images/math/2/9/2/292803eddd8e701579bdb8e0f9307a89.png)