From CFD-Wiki
2D case
For calculations in complex geometries boundary-fitted non-orthogonal curvlinear grids is usually used.
General transport equation is transformed from the physical domain  into the computational domain
into the computational domain 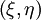 as the following equation
as the following equation
![\frac{\partial}{\partial \xi} \left( \rho U \phi \right) + \frac{\partial }{ \partial \eta } \left( \rho V \phi \right) = \frac{\partial}{\partial \xi} \left[ \frac{\Gamma }{J} \left( \alpha \frac{\partial \phi}{\partial \xi} - \beta \frac{\partial \phi}{ \partial \eta} \right) \right] + \frac{\partial}{\partial \eta} \left[ \frac{\Gamma}{J} \left( \gamma \frac{\partial \phi}{\partial \eta} - \beta \frac{\partial \phi}{ \partial \xi} \right) \right] + J S^{\phi}](/W/images/math/3/8/2/382456ea15e881910f1b040f17a347bd.png)
| (2) |
where
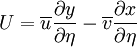
| (3) |
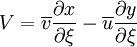
| (4) |
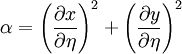
| (5) |
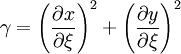
| (6) |
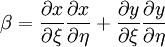
| (7) |
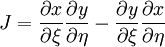
| (8) |
Using the finite volume method the trnsformed equations can be integrated as follows:
![\left[ \left( \rho U \Delta \eta \right) \right]^{e}_{w} + \left[ \left( \rho V \Delta \xi \right) \right]^{n}_{s} = \left[ \frac{\Gamma \Delta \eta}{J} \left( \alpha \frac{\partial \phi}{\partial \xi} - \beta \frac{\partial \phi}{ \partial \eta} \right) \right]^{e}_{w} + \left[ \frac{\Gamma \Delta \xi}{J} \left( \gamma \frac{\partial \phi}{\partial \eta } - \beta \frac{\partial \phi}{\partial \xi} \right) \right]^{n}_{s} + \left( J \Delta \xi \Delta \eta \right) \overline{S}^{\phi}_{P}](/W/images/math/c/9/b/c9bc323ba9595d8d85677016f4e53536.png)
| (9) |
The convection terms are approximated as described in section http://www.cfd-online.com/Wiki/Discretization_of_the_convection_term .
Diffusion terms are approximated by the second-oder central differencing scheme.
The standard form of the finite volume equation can be obtained as
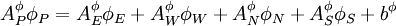
| (10) |
where
![A^{\phi}_{E} = \left(\frac{\Gamma}{J} \alpha \frac{\Delta \eta}{\Delta \xi} \right)_{e} + max \left[ 0, - \left( \rho U \Delta \eta \right)_{e} \right]](/W/images/math/d/d/3/dd391b55e6d5ab853dfb8a363e1ad14e.png)
| (11) |
![A^{\phi}_{W} = \left(\frac{\Gamma}{J} \alpha \frac{\Delta \eta}{\Delta \xi} \right)_{w} + max \left[ 0, \left( \rho U \Delta \eta \right)_{w} \right]](/W/images/math/0/d/b/0dbf08dd7c67f1ce5b898703ed51ba9e.png)
| (12) |
![A^{\phi}_{N} = \left( \frac{ \Gamma }{J} \gamma \frac{\Delta \xi}{\Delta \eta} \right)_{n} + max \left[ 0, - \left( \rho V \Delta \xi \right)_{n} \right]](/W/images/math/7/c/1/7c191e197bd433da99e710d3972cb506.png)
| (13) |
![A^{\phi}_{S} = \left( \frac{ \Gamma }{J} \gamma \frac{\Delta \xi}{\Delta \eta} \right)_{s} + max \left[ 0, \left( \rho V \Delta \xi \right)_{s} \right]](/W/images/math/3/8/3/383089c82dad52e5e81f6a38822a7428.png)
| (14) |
![b^{\phi} = \left( J \Delta \xi \Delta \eta \right) \overline{S}^{\phi}_{P} - \left[ \frac{\Gamma \Delta \eta}{J} \left( \beta \frac{\partial \phi}{\partial \eta} \right) \right]^{e}_{w} - \left[ \frac{\Gamma \Delta \xi}{J} \left( \beta \frac{\partial \phi}{\partial \xi} \right) \right]^{n}_{s}](/W/images/math/6/7/9/679e9d836ee26246cc251d1718a29333.png)
| (15) |
 into the computational domain
into the computational domain 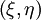 as the following equation
as the following equation

![\frac{\partial}{\partial \xi} \left( \rho U \phi \right) + \frac{\partial }{ \partial \eta } \left( \rho V \phi \right) = \frac{\partial}{\partial \xi} \left[ \frac{\Gamma }{J} \left( \alpha \frac{\partial \phi}{\partial \xi} - \beta \frac{\partial \phi}{ \partial \eta} \right) \right] + \frac{\partial}{\partial \eta} \left[ \frac{\Gamma}{J} \left( \gamma \frac{\partial \phi}{\partial \eta} - \beta \frac{\partial \phi}{ \partial \xi} \right) \right] + J S^{\phi}](/W/images/math/3/8/2/382456ea15e881910f1b040f17a347bd.png)
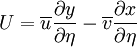
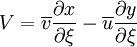
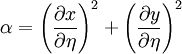
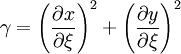
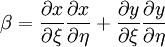
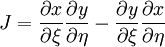
![\left[ \left( \rho U \Delta \eta \right) \right]^{e}_{w} + \left[ \left( \rho V \Delta \xi \right) \right]^{n}_{s} = \left[ \frac{\Gamma \Delta \eta}{J} \left( \alpha \frac{\partial \phi}{\partial \xi} - \beta \frac{\partial \phi}{ \partial \eta} \right) \right]^{e}_{w} + \left[ \frac{\Gamma \Delta \xi}{J} \left( \gamma \frac{\partial \phi}{\partial \eta } - \beta \frac{\partial \phi}{\partial \xi} \right) \right]^{n}_{s} + \left( J \Delta \xi \Delta \eta \right) \overline{S}^{\phi}_{P}](/W/images/math/c/9/b/c9bc323ba9595d8d85677016f4e53536.png)
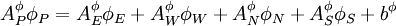
![A^{\phi}_{E} = \left(\frac{\Gamma}{J} \alpha \frac{\Delta \eta}{\Delta \xi} \right)_{e} + max \left[ 0, - \left( \rho U \Delta \eta \right)_{e} \right]](/W/images/math/d/d/3/dd391b55e6d5ab853dfb8a363e1ad14e.png)
![A^{\phi}_{W} = \left(\frac{\Gamma}{J} \alpha \frac{\Delta \eta}{\Delta \xi} \right)_{w} + max \left[ 0, \left( \rho U \Delta \eta \right)_{w} \right]](/W/images/math/0/d/b/0dbf08dd7c67f1ce5b898703ed51ba9e.png)
![A^{\phi}_{N} = \left( \frac{ \Gamma }{J} \gamma \frac{\Delta \xi}{\Delta \eta} \right)_{n} + max \left[ 0, - \left( \rho V \Delta \xi \right)_{n} \right]](/W/images/math/7/c/1/7c191e197bd433da99e710d3972cb506.png)
![A^{\phi}_{S} = \left( \frac{ \Gamma }{J} \gamma \frac{\Delta \xi}{\Delta \eta} \right)_{s} + max \left[ 0, \left( \rho V \Delta \xi \right)_{s} \right]](/W/images/math/3/8/3/383089c82dad52e5e81f6a38822a7428.png)
![b^{\phi} = \left( J \Delta \xi \Delta \eta \right) \overline{S}^{\phi}_{P} - \left[ \frac{\Gamma \Delta \eta}{J} \left( \beta \frac{\partial \phi}{\partial \eta} \right) \right]^{e}_{w} - \left[ \frac{\Gamma \Delta \xi}{J} \left( \beta \frac{\partial \phi}{\partial \xi} \right) \right]^{n}_{s}](/W/images/math/6/7/9/679e9d836ee26246cc251d1718a29333.png)