 |
|
|
[Sponsors] | |||||
|
|
|
#1 |
|
Senior Member
|
In section 7.3.3 of Pope's book on p.304, he derived the law of the wall based on the mixing-length hypothesis. Start with the total shear stress which is Eq.(7.142) in his book
 where  denotes quantities in wall units, denotes quantities in wall units,  is the mixing length, is the mixing length,  and and 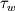 are the total and wall shear stress, respectively. are the total and wall shear stress, respectively. This is a quadratic equation for  , which should have solution , which should have solution  However, Pope gives a very different answer  This is very strange, I can't see where this is coming from. Does anybody have any idea? |
|
|
|

|
|
|
|
|
#2 |
|
Senior Member
|
Quote:
The Pope's formula, which is itself taken from the van Driest paper, remains valid also when the mixing length goes to 0, which is not the case for the standard formula. However, when both valid, they are equivalent, as you can see from the link. There may also be a reason linked to the stability of the formula, with the one in the book and paper being known also for its stability, but the validity for the mixing length equal 0 is the most prominent one in my opinion. |
|
|
|

|
|
 |
| Tags |
| boundary layers, law of the wall, van driest damping |
|
|
 Similar Threads
Similar Threads
|
||||
| Thread | Thread Starter | Forum | Replies | Last Post |
| [snappyHexMesh] How to draw a 3D-Drawing for Meshing | Kahnbein.Kai | OpenFOAM Meshing & Mesh Conversion | 4 | June 15, 2021 13:16 |
| Wall treatment with OpenFOAM | roby | OpenFOAM Running, Solving & CFD | 48 | May 28, 2021 12:38 |
| [Commercial meshers] Fluent3DMeshToFoam | simvun | OpenFOAM Meshing & Mesh Conversion | 50 | January 19, 2020 16:33 |
| Centrifugal fan | j0hnny | CFX | 13 | October 1, 2019 14:55 |
| [Commercial meshers] tmerge utility creates unwanted interface/walls comes in the final mesh | Shoonya | OpenFOAM Meshing & Mesh Conversion | 11 | January 20, 2012 07:23 |