 |
|
|
[Sponsors] | |||||
Linearization of the source term of the diffusion equation |
 |
|
|
LinkBack | Thread Tools | Search this Thread | Display Modes |
|
|
|
#1 |
|
New Member
Zhejiang
Join Date: Oct 2022
Posts: 2
Rep Power: 0  |
Hi guys,
I want to solve a convection-diffusion equation with an absorption source term (Liner Driving Force model and Langmuir-Freundlich isothermals), but I don't know how to linearise this source term. Please see the following equations: 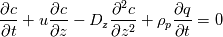 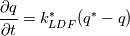 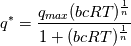 where c is the variable, and others are constant. I wonder how to linearise this 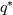 . Please give me some advice, and I really appreciate your time and patience. . Please give me some advice, and I really appreciate your time and patience.
|
|
|
|

|
|
|
|
|
#2 |
|
Senior Member
|
One typically writes the source term as a linearization around a 0 state (i.e., previous iteration value)
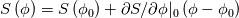 , but then the actual implementation depends from the specific method you are using (i.e., solving in delta form or not). See also https://www.cfd-online.com/Wiki/Sour..._linearization. , but then the actual implementation depends from the specific method you are using (i.e., solving in delta form or not). See also https://www.cfd-online.com/Wiki/Sour..._linearization.Hopefully, you can do 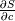 by yourself, but the possibly problematic point I see here is that the derivative is not defined at c=0 (but the source term is). Honestly, I never dealed with such problem, but a possible way out is to simply saturate the derivative at a minimum value of c (but, again, this might depend from the implemetation). by yourself, but the possibly problematic point I see here is that the derivative is not defined at c=0 (but the source term is). Honestly, I never dealed with such problem, but a possible way out is to simply saturate the derivative at a minimum value of c (but, again, this might depend from the implemetation).
|
|
|
|

|
|
|
|
|
#3 |
|
New Member
Zhejiang
Join Date: Oct 2022
Posts: 2
Rep Power: 0  |
Thank you for your reply, I will read the web you mentioned.
|
|
|
|

|
|
 |
| Tags |
| absorptivity, diffusion equation, linearize, source terms |
|
|
 Similar Threads
Similar Threads
|
||||
| Thread | Thread Starter | Forum | Replies | Last Post |
| Using PengRobinsonGas EoS with sprayFoam | Jabo | OpenFOAM Running, Solving & CFD | 36 | July 16, 2024 04:52 |
| [swak4Foam] funkyDoCalc with OF2.3 massflow | NiFl | OpenFOAM Community Contributions | 14 | November 25, 2020 04:30 |
| [swak4Foam] swak4foam building problem | GGerber | OpenFOAM Community Contributions | 54 | April 24, 2015 17:02 |
| friction forces icoFoam | ofslcm | OpenFOAM | 3 | April 7, 2012 11:57 |
| OpenFOAM on MinGW crosscompiler hosted on Linux | allenzhao | OpenFOAM Installation | 127 | January 30, 2009 20:08 |