 |
|
|
[Sponsors] | |||||
|
|
|
#1 |
|
Senior Member
Join Date: Dec 2017
Posts: 153
Rep Power: 8  |
Hello,
Today I have a question for you regarding TVD schemes on non-uniform meshes. Starting from the uniform case, we know that the face value of a general variable can be recovered using the following formula:  where: D= downwind node C= upwind node U = second upwind node r=  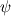 = flux limiter = flux limiterSo if the limiter is zero then we have upwind, while if the limite is 1 we have a central difference scheme. Great! If the grid is non-uniform,  does not mean CDS, this is because the 1/2 in front of the limiter should be substituted by the classical interpolation weight factor does not mean CDS, this is because the 1/2 in front of the limiter should be substituted by the classical interpolation weight factor  and this adjusts the things. and this adjusts the things.Now here comes my question: consider the Van Leer flux limiter (at least what i have found around the web): (  its limit for r to infty is 2. However, for a non-uniform mesh we have:  , , and so the downwind scheme is not recovered in the limit case of very large r as should be... Can somebody shed light on this behaviour? Maybe the limiter expression holds only for uniform grids? Thank you very much, AliE Last edited by AliE; December 28, 2017 at 15:04. |
|
|
|

|
|
|
|
|
#2 |
|
Senior Member
Michael Prinkey
Join Date: Mar 2009
Location: Pittsburgh PA
Posts: 363
Rep Power: 25  |
In my experience, most discussion of limiters on unevenly (and even unstructured) methods are better considered in the context of gradient limiters. I'd refer you to the seminal paper on the subject, Barth, T. and Jespersen, D., “The design and application of upwind schemes on unstructured meshes,” AIAA-89-0366, 1989.But, IMO Marsha Berger does a better overview of the topic in a semi-review paper here:
https://www.nas.nasa.gov/assets/pdf/...nas-05-007.pdf It does a nice job of bridging the gap and justifying the rationale of gradient limiting versus the canonical explicit forms used for evenly spaces methods. Good luck. |
|
|
|

|
|
 |
| Tags |
| limiters, tvd |
|
|
 Similar Threads
Similar Threads
|
||||
| Thread | Thread Starter | Forum | Replies | Last Post |
| TVD schemes, questions about limitedLinear | davibarreira | OpenFOAM | 31 | October 21, 2021 00:05 |
| boundedness of TVD in 3D unstructured grids | Lydia | OpenFOAM Programming & Development | 4 | July 9, 2014 17:31 |
| TVD schemes | Vino | Main CFD Forum | 3 | September 16, 2013 20:47 |
| TVD schemes: 2 questions | Shuo | Main CFD Forum | 0 | August 19, 2008 22:51 |
| TVD Schemes | Matt Umbel | Main CFD Forum | 1 | January 25, 2000 05:21 |