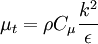Realisable k-epsilon model
From CFD-Wiki
(Difference between revisions)
| Line 12: | Line 12: | ||
In these equations, <math> P_k </math> represents the generation of turbulence kinetic energy due to the mean velocity gradients, calculated in same manner as standard k-epsilon model. <math> P_b </math> is the generation of turbulence kinetic energy due to buoyancy, calculated in same way as standard k-epsilon model. | In these equations, <math> P_k </math> represents the generation of turbulence kinetic energy due to the mean velocity gradients, calculated in same manner as standard k-epsilon model. <math> P_b </math> is the generation of turbulence kinetic energy due to buoyancy, calculated in same way as standard k-epsilon model. | ||
| + | |||
| + | == Modelling Turbulent Viscosity == | ||
| + | |||
| + | :<math> \mu_t = \rho C_{\mu} \frac{k^2}{\epsilon} </math> <br> | ||
| + | where <br> | ||
| + | <math> C_{\mu} = \frac{1}{A_0 + A_s \frac{k U^*}{\epsilon}} </math> <br> | ||
| + | <math> U^* \equiv \sqrt{S_{ij} S_{ij} + \tilde{\Omega}_{ij} \tilde{\Omega}_{ij}} </math> ;<br> | ||
| + | <math> \tilde{\Omega}_{ij} = \Omega_{ij} - 2 \epsilon_{ijk} \omega_k </math> ; <br> | ||
| + | <math> \Omega_{ij} = \overline{\Omega_{ij}} - \epsilon_{ijk} \omega_k </math> <br> | ||
| + | |||
| + | where <math> \overline{\Omega_{ij}} </math> is the mean rate-of-rotation tensor viewed in a rotating reference frame with the angular velocity <math> \omega_k </math>. The model constants <math> A_0 </math> and <math> A_s </math> are given by: <br> | ||
| + | <math> A_0 = 4.04, \; \; A_s = \sqrt{6} \cos \phi </math> | ||
Revision as of 23:06, 18 September 2005
Transport Equations
Where
![C_1 = \max\left[0.43, \frac{\eta}{\eta + 5}\right] , \;\;\;\;\; \eta = S \frac{k}{\epsilon}, \;\;\;\;\; S =\sqrt{2 S_{ij} S_{ij}}](/W/images/math/1/9/3/193f62d995a6b0190d41325b612bf336.png)
In these equations, 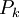 represents the generation of turbulence kinetic energy due to the mean velocity gradients, calculated in same manner as standard k-epsilon model.
represents the generation of turbulence kinetic energy due to the mean velocity gradients, calculated in same manner as standard k-epsilon model. 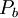 is the generation of turbulence kinetic energy due to buoyancy, calculated in same way as standard k-epsilon model.
is the generation of turbulence kinetic energy due to buoyancy, calculated in same way as standard k-epsilon model.
Modelling Turbulent Viscosity
where
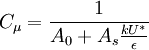
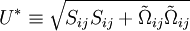 ;
;
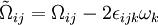 ;
;
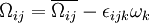
where 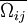 is the mean rate-of-rotation tensor viewed in a rotating reference frame with the angular velocity
is the mean rate-of-rotation tensor viewed in a rotating reference frame with the angular velocity 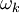 . The model constants
. The model constants  and
and 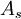 are given by:
are given by:
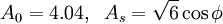

![\frac{\partial}{\partial t} (\rho k) + \frac{\partial}{\partial x_j} (\rho k u_j) = \frac{\partial}{\partial x_j} \left [ \left(\mu + \frac{\mu_t}{\sigma_k}\right) \frac{\partial k} {\partial x_j} \right ] + P_k + P_b - \rho \epsilon - Y_M + S_k](/W/images/math/0/0/4/004f9597d5f640c6152d7ee7ec2af16a.png)
![\frac{\partial}{\partial t} (\rho \epsilon) + \frac{\partial}{\partial x_j} (\rho \epsilon u_j) = \frac{\partial}{\partial x_j} \left[ \left(\mu + \frac{\mu_t}{\sigma_{\epsilon}}\right) \frac{\partial \epsilon}{\partial x_j} \right ] + \rho \, C_1 S \epsilon - \rho \, C_2 \frac{{\epsilon}^2} {k + \sqrt{\nu \epsilon}} + C_{1 \epsilon}\frac{\epsilon}{k} C_{3 \epsilon} P_b + S_{\epsilon}](/W/images/math/9/e/1/9e195cf17f086a6a1ad3914571b491ba.png)