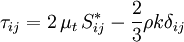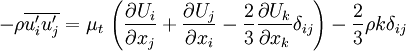CFD Online Logo CFD Online URL
www.cfd-online.com
[Sponsors]
Home > Wiki > Boussinesq eddy viscosity assumption

Boussinesq eddy viscosity assumption

From CFD-Wiki

(Difference between revisions)
Jump to: navigation, search
m
m (Reverted edits by Reverse22 (talk) to last revision by Peter)
 
(3 intermediate revisions not shown)

Latest revision as of 09:15, 3 January 2012

In 1877 Boussinesq postulated[1][2] that the momentum transfer caused by turbulent eddies can be modeled with an eddy viscosity. This is in analogy with how the momentum transfer caused by the molecular motion in a gas can be described by a molecular viscosity. The Boussinesq assumption states that the Reynolds stress tensor, 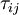\tau_{ij}, is proportional to the trace-less mean strain rate tensor, 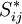S_{ij}^*, and can be written in the following way:

\tau_{ij} = 2 \, \mu_t \, S_{ij}^* - \frac{2}{3} \rho k \delta_{ij}

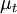Where \mu_t is a scalar property called the eddy viscosity. The same equation can be written more explicitly as:

-\rho \overline{u'_i u'_j} = \mu_t \, \left( \frac{\partial U_i}{\partial x_j} + \frac{\partial U_j}{\partial x_i} - \frac{2}{3} \frac{\partial U_k}{\partial x_k} \delta_{ij} \right) - \frac{2}{3} \rho k \delta_{ij}

Note that for incompressible flow:

\frac{\partial U_k}{\partial x_k} = 0

The Boussinesq eddy viscosity assumption is also often called the Boussinesq hypothesis or the Boussinesq approximation.

See also

References

  1. ↑ Boussinesq, J. (19877), "Essai sur la théorie des eaux courantes", Mémoires présentés par divers savants à l'Académie des Sciences 23 (1): 1-680
  2. ↑ Schmitt, F.G. (2007), "About Boussinesq’s turbulent viscosity hypothesis: historical remarks and a direct evaluation of its validity", Comptes Rendus Mécanique 335 ((9-10)): 617-627


This article is a stub, a short article which needs to be improved. You can help by expanding it.
Retrieved from "https://cfd-online.com/Wiki/Boussinesq_eddy_viscosity_assumption"
My wiki