 |
|
|
[Sponsors] | |||||
|
|
|
#1 |
|
Senior Member
|
http://www.kxcad.net/ansys/ANSYS_CFX.../i1311648.html
It is stated in the CFX theory (above link) that when one selects the high resolution scheme as below 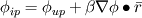 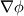 is the value at the upwind node. is the value at the upwind node.On the other hand when user selects the specified blend factor for 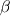 (between 0 and 1), (between 0 and 1), 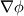 is equal to the average of the adjacent nodal gradients. I wanna know, this scheme is the upwind or central differencing scheme? is equal to the average of the adjacent nodal gradients. I wanna know, this scheme is the upwind or central differencing scheme?http://my.fit.edu/itresources/manual...ug/node992.htm Where as in fluent user guide (above link) 2nd order upwind scheme is given by following formula 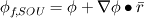 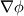 is the gradient of is the gradient of 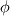 in the upwind cell in the upwind cellBoth high resolution (CFX) and 2nd order upwind scheme (Fluent) are based on the principles by Barth and Jespersen [1] so that no new extrema is introduced in the solution, therfore monotonic behavior is preserved. 1. Does it mean that the high resolution scheme of CFX and 2nd order upwind scheme of fluent are equivalent. 2. Does it mean that the CFX 2nd order scheme is more like a baised 2nd order scheme with one term of upwind and 2nd term (anti diffusive term) is central differencing type? 3. Will 2nd order upwind (CFX definition) will make the solution worst than even 1st order upwind scheme? References: [1] Barth and Jespersen "The design and application of upwind schemes on unstructured meshes" . Technical Report AIAA-89-0366, AIAA 27th Aerospace Sciences Meeting, Reno, Nevada, 1989. |
|
|
|

|
|
|
|
|
#2 | |
|
Senior Member
|
Hello,
phi_ip = phi_up - based on flux direction is upwind. Anything added to it, makes high resolution. Central diff simply takes 0.5 as blending factor. Different schemes like bounded TVD schemes are all based on how to manipulate the blending factors to give a variable value bounded between up and downwind cells. Upwind schemes are most stable irrespective of meshes..Central is accurate but not stable..one can stabilize by dynamically varying the blending factors based on certain functions of the flow. I think you shouldnt worry about comparing "solutions" from one black box to another ! Simply should rather focus on what fundamentally the schemes generate. Again, any other terms than phi_up, in calculating phi_ip, is already away from upwind ! Good luck. CFDToy Quote:
__________________
CFDtoy |
||
|
|

|
||
|
|
|
#3 | ||
|
Senior Member
|
Quote:
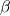 = 1 scheme is 2nd order unwind (correct me if I am wrong) = 1 scheme is 2nd order unwind (correct me if I am wrong)Quote:
http://www.cfd-online.com/Forums/cfx...machinery.html More over we have results which have very different trends with spalart allmars model. Here I my task is to find that why results are varying (see above link). Is this due to mesh quality, advection scheme, missing term in spalart allamras model or due to solver implementation. In fact we have very large data base and we are working on it for last three years. For example we have created meshes with yplus = 1, 2.5, 5, 10, 20, 30, 40 , 60 and 80 and testing the different turbulence model specially SA model. We are also assessing boundary conditions I hope now you realize why it is important for me to understand the different aspects of solution |
|||
|
|

|
|||
|
|
|
#5 |
|
Member
Max
Join Date: May 2011
Location: old europe
Posts: 88
Rep Power: 15  |
Another questions concerning these schemes:
The the theory guide states that 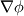 is the upwind gradient. But how is it calculated? is the upwind gradient. But how is it calculated? In my work, the advection scheme is of major importance and i want to express  in terms of nodal values of phi. Like Far already stated, in terms of nodal values of phi. Like Far already stated,  results in 2nd order upwind. I guess that leads (for simplification in a 1-dimensional case) to: results in 2nd order upwind. I guess that leads (for simplification in a 1-dimensional case) to: with with  being the second node in upstream direction and being the second node in upstream direction and  beeing the distance between two nodes. See, figure: beeing the distance between two nodes. See, figure:http://imageshack.us/photo/my-images/401/highresx.jpg/ Or am I totally confusing something? I'm do not have a real insight into finite differencing yet. I hope somebody can help me. Thanks in Advance! Last edited by wyldckat; September 3, 2015 at 19:05. Reason: disabled embedded images |
|
|
|

|
|
|
|
|
#6 |
|
New Member
kian
Join Date: Feb 2013
Posts: 14
Rep Power: 13  |
Hi, I've got a fortran code for solving 2D compressible flow over an airfoil with the method of first order van leer flux vector splitting . I have to change it to second order van leer flux vector splitting method . Can you please help me on how to do it? It's also possible for me to solve this project with the method of AUSM+ . Are you familiar with this method? can you help me with it please?
Thanks a lot in advance |
|
|
|

|
|
|
|
|
#7 | |
|
Senior Member
duri
Join Date: May 2010
Posts: 245
Rep Power: 17  |
Quote:
Upwind or central scheme dictates the face flux estimation. In cfx manual its is not clear that how face values are estimated. It can be pure central scheme like 0.5(UL+UR) or upwind like UL or UR can be partly central and partly upwind (eg., pressure central and velocity upwind like in AUSM). Quote:
not necessarily, but if first order behaviour of both the schemes are same then both are equivalent. Second order is just extension of first order with better reconstruction. Quote:
I couldn't understand 2nd question. 2nd order upwind scheme with insufficient limiter can make solution worse near large gradients. |
||
|
|

|
||
|
|
|
#8 |
|
New Member
kian
Join Date: Feb 2013
Posts: 14
Rep Power: 13  |
Thanks a lot for your comment
|
|
|
|

|
|
 |
|
|
 Similar Threads
Similar Threads
|
||||
| Thread | Thread Starter | Forum | Replies | Last Post |
| 2nd order upwind and QUICK scheme | Wiroon | Main CFD Forum | 19 | September 17, 2019 04:47 |
| 1st order vs 2nd order | ken | FLUENT | 8 | March 14, 2013 04:43 |
| Second order upwind is not UPwind!!! | Far | CFX | 9 | May 31, 2011 09:21 |
| 2nd order upwind scheme (Fluent and CFX) | Far | FLUENT | 0 | May 22, 2011 02:50 |
| Changing LimitedLinear to blend with 2nd order upwind instead of 1st order upwind | stevenvanharen | OpenFOAM Programming & Development | 0 | April 11, 2011 06:54 |