 October 19, 2020, 17:22
October 19, 2020, 17:22
|
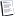 2nd-order TVD criteria for flux limiter methods
2nd-order TVD criteria for flux limiter methods
|
#1
|
|
New Member
Join Date: Oct 2020
Posts: 1
Rep Power: 0
|
Hello, this is my first question here
I have a system of 1D nonlinear conservative hyperbolic equations which will form shocks after finite time. I'd like to evolve them via method-of lines (MOL) with a flux limiter for the high- and low-order spatial fluxes.
Most sources simply state that to keep the overall method 2nd-order and TVD, the limiter function must lie in the region given by the attached image:

I was able to derive the same conditions for the overall TVD region, but I do not understand where the blue region comes from. I have obtained a copy of the original paper by Sweby (1984) but it isn't clear to me at all. Also, his methods are based on Lax-Wendroff as a starting point. How can I be sure these conditions generalize to any choice of 2nd-order method, such as central difference? I was under the impression that you could pick and choose any purely spatial higher order scheme and apply the limiter in conjunction with 1st-order upwind.
I have asked this question also on stackexchange without much luck, so for those who have an account there you can earn some rep by answering:
http://https://scicomp.stackexchange...r-flux-limiter
|

|

|






 Similar Threads
Similar Threads