2d SWE -- CFD Online Discussion Forums

August 24, 2016, 08:04	2d SWE	#1
FrancescoLRomeo New Member Francesco L. Romeo Join Date: Jun 2016 Location: Milan, Italy Posts: 6 Rep Power: 10	Hi, I have some questions about the 2D SWE (Shallow Water Equations). I would like to know if an exact (analytical) solution is available in the case of the (linear) surface "gravity" wave. Indeed, my example of interest is given by a square , with initial velocity field 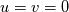and an initial Gaussian perturbation of the free surface elevation placed in the center of the domain: $h(x,y,t=0) = H + \eta' \exp \biggl[- \frac{ (x - L/2)^2 }{2 \sigma_x^2} \biggl] \exp \biggl[- \frac{ (y - L/2)^2 }{2 \sigma_y^2} \biggl]$ I know that, in 1D, a simple exact solution is available, which derives from a linearization of the Sain-Venant equations. Can you give me some references? (I am studying on Leveque, "Finite Volume methods for hyperbolic Problems" 2004) Thank you, Best regards, Francesco

Tags

Posting Rules
You may not post new threads You may not post replies You may not post attachments You may not edit your posts BB code is On Smilies are On [IMG] code is On HTML code is Off Trackbacks are Off Pingbacks are On Refbacks are On Forum Rules

All times are GMT -4. The time now is 11:15.