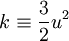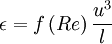Introduction to turbulence/Homogeneous turbulence
From CFD-Wiki
A first look at decaying turbulence
Look, for example, at the decay of turbulence which has already been generated. If this turbulence is homogeneous and there is no mean velocity gradient to generate new turbulence, the kinetic energy equation reduces to simply:
|
| (1) |
This is often written (especially for isotropic turbulence) as:
|
| (2) |
where
|
| (3) |
Now you can't get any simpler than this. Yet unbelievably we still don't have enough information to solve it. Let's try. Suppose we use the extanded ideas of Kolmogorov we introduced in Chapter 3 to related the dissipation to the turbulence energy, say:
|
| (4) |
Already you can see we have two problems, what is 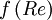 , and what is the time dependece of
, and what is the time dependece of 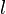 ? Now there is practically a different answer to these questions for every investigator in turbulence - most of whom will assure you their choice is only reasonable one.
? Now there is practically a different answer to these questions for every investigator in turbulence - most of whom will assure you their choice is only reasonable one.
Figure 6.1 shows an attempt to correlate some of the grid turbulence data using the longitudinal integral scale for 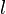 , i.e.,
, i.e., 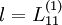 , or simply
, or simply 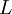 . The first thing you notice is the problem at low Reynolds number. The second is probably the possible asymptote at the higher Reynolds numbers.
. The first thing you notice is the problem at low Reynolds number. The second is probably the possible asymptote at the higher Reynolds numbers.

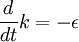
![\frac{d}{dt} \left[ \frac{3}{2} u^{2} \right] = - \epsilon](/W/images/math/a/c/2/ac251958c2df0a8a260f8f83d2645da2.png)