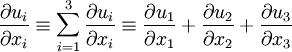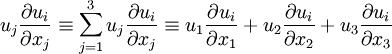Einstein summation convention
From CFD-Wiki
The Einstein summation convention is a tensor notation which is commonly used to implicitly define a sum. The convention states that when an index is repeated in a term that implies a sum over all possible values for that index.
Here are two examples: