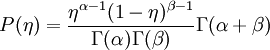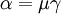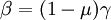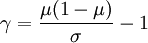Beta PDF
From CFD-Wiki
A 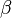 probability density function depends on
two moments only; the mean
probability density function depends on
two moments only; the mean 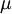 and the variance
and the variance 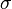 .
This function is widely used in turbulent combustion to define the scalar distribution at each
computational point as a function of the mean and variance.
Assuming that the sample space of the scalar varies betwen 0 and 1.
The beta function PDF has the form
.
This function is widely used in turbulent combustion to define the scalar distribution at each
computational point as a function of the mean and variance.
Assuming that the sample space of the scalar varies betwen 0 and 1.
The beta function PDF has the form
where 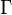 is the gamma function and the parameters
is the gamma function and the parameters
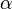 and
and 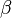 are related through
are related through
where 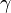 is
is