Approximation Schemes for convective term - structured grids - Summary of Discretizations Schemes and examples
From CFD-Wiki
(→Example code for solving Smith-Hutton test) |
(→Example code for solving Smith-Hutton test) |
||
| Line 38: | Line 38: | ||
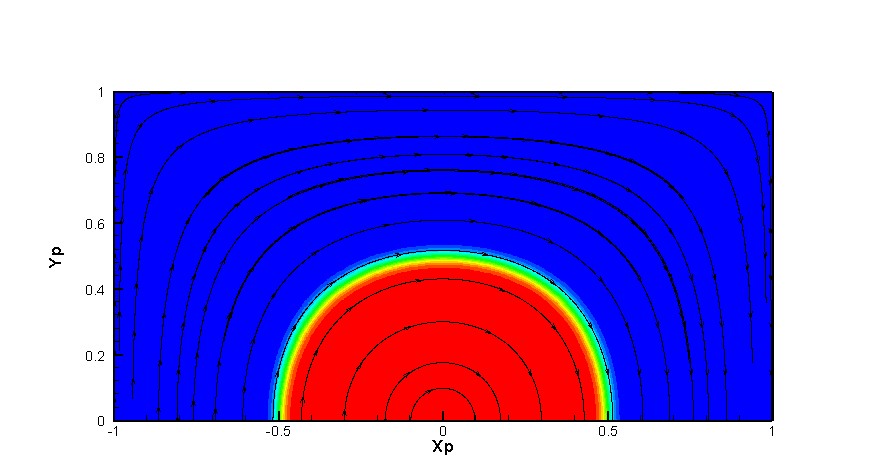
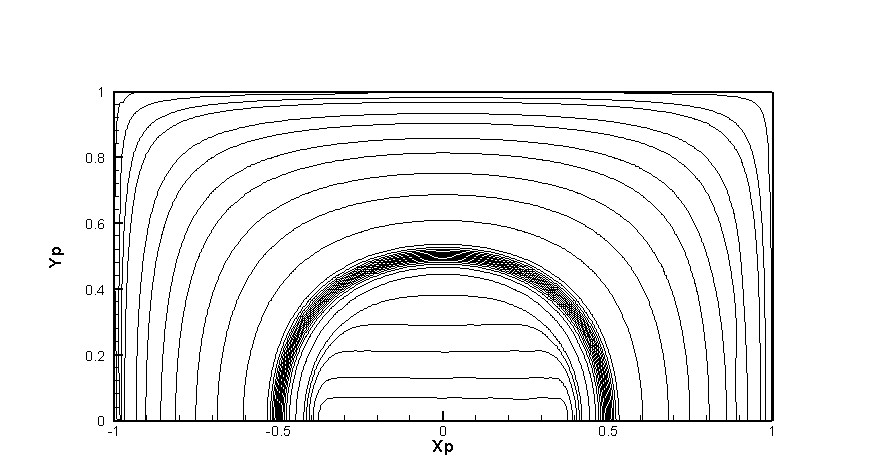
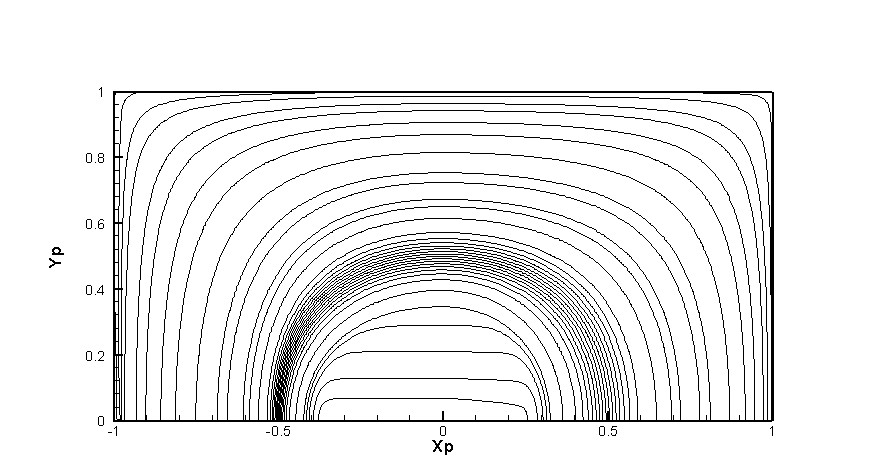
It's a results, obtained using this code ('''UDS''' and '''HLPA''' schemes) | It's a results, obtained using this code ('''UDS''' and '''HLPA''' schemes) | ||
| + | Below it's cleary seen the diffusion impact, comparing the contour fields obtaining using the UDS and HLPA. A bit later we shall place here a solution gained with QUICK scheme, and it will be seen the osscilations. | ||
[[Image:NM_convectionschemes_struct_grids_Smith_Huton_Vector_Field_probe_01.jpg]] | [[Image:NM_convectionschemes_struct_grids_Smith_Huton_Vector_Field_probe_01.jpg]] | ||
Revision as of 16:16, 22 September 2005
When we shall fill this page, I offer to make common identifications, because in different issues was used different notation.
Also we beg everybody to help me with original works. Later I shall write, what is necessary. If anyone have literature connected with convective schemes, please drop me a line.
We shall be very glad and grateful to hear any critical suggestion (please drop a few lines at Wiki Forum)
It is just a skeleton, but we hope that it will be developed into the good thing
Contents |
Discretizations Schemes Estimation of order
Discretizations Schemes Estimation of error
Selection advice
Comparison of Discretizations Schemes
Numerical examples
Pure convection of a scalar step by a rotating velocity field (Smith-Hutton test)
R.M.Smith and A.G.Hutton (1982), "The numerical treatment of advection: A performance comparison of current methods", Numerical Heat Transfer, Vol. 5, p439.
Square Lid-driven cavity flow
Example code for solving Smith-Hutton test
Dear friends
It's just a scrap. Later I'll correct it, although it's a complete working code
Michail
Sample code for solving Smith-Hutton test - Fortran 90
It's a results, obtained using this code (UDS and HLPA schemes)
Below it's cleary seen the diffusion impact, comparing the contour fields obtaining using the UDS and HLPA. A bit later we shall place here a solution gained with QUICK scheme, and it will be seen the osscilations.